Youtube daily report Oct 4 2017

Rain, rain, go away

Come again another day

Daddy wants to play

Rain, rain, go away

Rain, rain, go away

Come again another day

Mommy wants to play

Rain, rain, go away

Rain, rain, go away

Come again another day

Little brother wants to play

Rain, rain, go away

Rain, rain, go away

Come again another day

Little sister wants to play

Rain, rain, go away

Rain, rain, go away

Come again another day

Baby wants to play

Rain, rain, go away

Rain, rain, go away

Come again another day

The family wants to play

Rain, rain, go away

For more infomation >> Lluvia Lluvia Vete Ya y Muchas Más Canciones Infantiles - Duration: 1:10:52.

-------------------------------------------

Graphing Lines in Algebra: Understanding Slopes and Y-Intercepts - Duration: 6:52.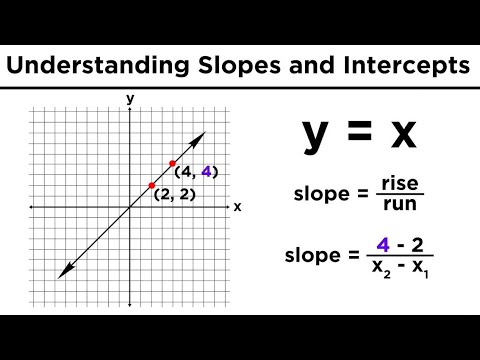

Professor Dave again, let's talk about slopes and intercepts.

In math, when we use the word slope, it doesn't mean we are going to hit the slopes, although

there is some similarity between the downward slope of a mountain and the meaning of this

word in math.

It means a line's rate of change in the vertical direction, which can be shallow or

steep, just like a mountain.

To understand how the slope of a line relates to the equation of the line, let's introduce

a common form for linear equations, Y equals MX plus B.

We already know about X and Y, these are the independent variable and dependent variable

respectively.

But these other two terms represent characteristics of the line.

M represents the slope of the line, which is as we said, essentially how steep the line

is, and B represents the Y-intercept, which is the Y coordinate for the point where the

line crosses the Y axis.

Let's start by looking at the simplest example possible, Y equals X.

We already learned how to plot this line, so here it is, with every point showing an

ordered pair where the X and Y values are equivalent.

To calculate the slope, we must understand that this is equal to rise over run, which

means change in Y over change in X.

We can pick any two points on this line, and with these points we take the difference in

their Y values, and divide by the difference in their X values.

Y is up and down, or the rise, and X is side to side, or the run.

Rise over run.

Let's pick these two points, two two and four four.

Rise over run can be represented this way, Y two minus Y one over X two minus X one,

so we just plug the coordinates in.

Y two is four, Y one is two, X two is four, and X one is two.

We simplify and get two over two, or one.

The slope of this line is one.

That should make sense, because Y equals X is in Y equals MX plus B form, it's just

that M is one and B is zero.

One X is just X, and B is zero, because the line crosses the Y axis when Y equals zero,

so we get this very simple equation, Y equals X.

If a line has a slope that is greater than one, it will tilt upwards like this, steeper

and steeper, climbing towards infinity, until it reaches complete verticality, at which

point the slope is undefined, because the line rises all the way to infinity in the

Y direction without any run at all, and infinity over zero is undefined.

If instead the slope is less than one, the line tilts downwards this way, the slope getting

smaller and smaller, until it is completely horizontal, at which point the slope will

become zero.

The line runs all the way to infinity in the X direction without rising at all, and zero

over infinity is zero.

So vertical lines have an undefined slope, horizontal lines have zero slope, and everything

in between has some slope between zero and infinity.

If we continue past the horizontal, the slope becomes increasingly negative, because as

we run in the positive direction, the rise is negative, or moving downwards.

The slope will increase in the negative direction, approaching negative infinity until vertical,

where the slope becomes undefined.

Let's just quickly mention that any two points define a line.

You could draw any two points at random on the coordinate plane, and there is a line

that connects them.

However, all lines contain an infinite number of points, since the coordinates can become

infinitely precise, and any point on the line represents an X and Y value that qualifies

as a solution to the equation.

We could pick two points, like three two and five seven, and draw a line between them.

We could also calculate the slope.

Seven minus two is five, and five minus three is two, so the slope is five halves.

Always remember that when calculating slope, it doesn't matter which point is point one

and which is point two, but the coordinates of each point must remain together, so whichever

Y value is Y two, the X value in that point must be X two, and not X one.

That would lead to an incorrect slope calculation.

Also, one point and a slope can define a line.

We could say that a line passes through two four and has a slope of three halves.

To draw this line, we would just go to the the point two four, and start applying the

slope.

Up three, over two.

This could also be down three, two the left, because negative three over negative two also

equals three halves, and that's how the slope, which we can regard as the rate of

change, makes sense regardless of which direction you travel.

Now that we understand slopes and Y intercepts, let's check comprehension.

For more infomation >> Graphing Lines in Algebra: Understanding Slopes and Y-Intercepts - Duration: 6:52.

-------------------------------------------

[VIDEO 2 de 3] - Curso de Oratoria y de Cómo Hablar en Público | TecnicasHablarEnPublico.com - Duration: 15:01.

For more infomation >> [VIDEO 2 de 3] - Curso de Oratoria y de Cómo Hablar en Público | TecnicasHablarEnPublico.com - Duration: 15:01.

-------------------------------------------

Nagore Robles y Sandra Barneda disfrutan sus vacaciones más exóticas en Tailandia - Duration: 2:04.

For more infomation >> Nagore Robles y Sandra Barneda disfrutan sus vacaciones más exóticas en Tailandia - Duration: 2:04.

-------------------------------------------

CONCURSO MAQUINATE! 2 | TU Y YO 😍 - Duration: 1:31.

For more infomation >> CONCURSO MAQUINATE! 2 | TU Y YO 😍 - Duration: 1:31.

-------------------------------------------

Sabino y Japo - Misión suicida - Duration: 3:05.

For more infomation >> Sabino y Japo - Misión suicida - Duration: 3:05.

-------------------------------------------

5 trucos para doblar la ropa y tener más espacio en tu armario - Duration: 9:16.

For more infomation >> 5 trucos para doblar la ropa y tener más espacio en tu armario - Duration: 9:16.

-------------------------------------------

iPhone 8 - Replacing the screen (iDoc repair guide) - Duration: 13:30.

For more infomation >> iPhone 8 - Replacing the screen (iDoc repair guide) - Duration: 13:30.

-------------------------------------------

Peppa Pig George m&m Chocolates Finger Family Song Colors Learn - Duration: 1:32.

Peppa Pig George m&m Chocolates Finger Family Song Colors Learn

For more infomation >> Peppa Pig George m&m Chocolates Finger Family Song Colors Learn - Duration: 1:32.

-------------------------------------------

My Little Pony Movie 2017 | Meu Pequeno Pônei Filme - Duration: 3:43.

My Little Pony Movie 2017 | Meu Pequeno Pônei Filme

For more infomation >> My Little Pony Movie 2017 | Meu Pequeno Pônei Filme - Duration: 3:43.

-------------------------------------------

Sherri's Sacred Story - Duration: 2:02.

Hi.

My name is Sherri Boggs.

I'm the Quality Patient Safety Manager

at Our Lady of Peace Hospital in Louisville, Kentucky,

a part of Kentucky One Health.

I have been a nurse for 22 years, all of those years

in the field of psychiatric mental health nursing.

Recently, I was able to participate

in my behavioral health hospital's Patient Family

and Advisory Council.

It was at this meeting that I was first introduced

to our newest committee member, who talked to us

and introduced himself as being a former patient

at our behavioral health hospital as an adolescent.

He told us of his story and how, for the first time,

someone was able to connect truly with him.

He said, this place saved my life.

The nurses and treatment team and doctor

saw me as a person, not as a mental health diagnosis.

He talked about how the doctor brought him to his office,

brushed his medical record off his desk, and said,

I'm not gonna learn about you through reading this.

I want you to talk about you.

He said, for the first time ever,

he was given the opportunity to truly be

involved in his own treatment.

And this led him on the road to recovery.

Now, as an adult, he is the successful CEO

helping others on their road to recovery working

at a nonprofit organization to get people on the road

to recovery and to break the cycle of poverty.

This former patient really was able to tell us

how important it is to truly listen and how in doing so,

we can connect person to person.

Have you ever had the opportunity

to truly listen to someone and to be listened to?

How important is that for each of us to do for our patients?

For more infomation >> Sherri's Sacred Story - Duration: 2:02.

-------------------------------------------

6 propiedades curativas del té de kombucha que no te puedes perder - Duration: 8:44.

For more infomation >> 6 propiedades curativas del té de kombucha que no te puedes perder - Duration: 8:44.

-------------------------------------------

Star Wars: Legión - Duration: 1:00.

For more infomation >> Star Wars: Legión - Duration: 1:00.

-------------------------------------------

Relaxing Flute: Beautiful Flute Melodies To Calm Thoughts & Relax - Duration: 1:02:11.

Relaxing Flute: Beautiful Flute Melodies To Calm Thoughts & Relax

For more infomation >> Relaxing Flute: Beautiful Flute Melodies To Calm Thoughts & Relax - Duration: 1:02:11.

-------------------------------------------

Piqué no renuncia a la selección española - Duration: 2:48.

For more infomation >> Piqué no renuncia a la selección española - Duration: 2:48.

-------------------------------------------

3 Step Formula to Making "Display Advertising" Profitable (So You Can Make More Money Online) - Duration: 2:07.

For more infomation >> 3 Step Formula to Making "Display Advertising" Profitable (So You Can Make More Money Online) - Duration: 2:07.

-------------------------------------------

DJI — Reflections — Oct 11, 2017 - Duration: 0:46.

What is it that allows us to dream,

to fear,

to feel?

Every flicker of light reflects the world around us

and makes us who we are.

Our minds will not compromise

but keep on wandering

looking for the images that reach our hearts.

The eternal tension between the creator and his creation.

That's what gives us the desire to strive for perfection

in all that we do.

For more infomation >> DJI — Reflections — Oct 11, 2017 - Duration: 0:46.

-------------------------------------------

Metáfora de la cinta transportadora de Sushi (observar pensamientos) de Russ Harris - Duration: 2:10.

For more infomation >> Metáfora de la cinta transportadora de Sushi (observar pensamientos) de Russ Harris - Duration: 2:10.

-------------------------------------------

Conoce a Isidora Aguirre - Ganadora EF Challenge 2017 - Duration: 1:04.

For more infomation >> Conoce a Isidora Aguirre - Ganadora EF Challenge 2017 - Duration: 1:04.

-------------------------------------------

El baile como herramienta solidaria - Duration: 2:21.

For more infomation >> El baile como herramienta solidaria - Duration: 2:21.

-------------------------------------------

Catch the mother of all news t...

For more infomation >> Catch the mother of all news t...

-------------------------------------------

Entertainment News 247 - マンU本拠地"夢の劇場"、拡張計画が判明…英最大級8.8万人収容へ増築か - Duration: 2:07.

For more infomation >> Entertainment News 247 - マンU本拠地"夢の劇場"、拡張計画が判明…英最大級8.8万人収容へ増築か - Duration: 2:07.

-------------------------------------------

Chính thức : Giải hạng 2 Kimpt Fifa Online 3 Championship - Vòng 2 - Mùa giải 2017/2018 - Duration: 2:05:22.

For more infomation >> Chính thức : Giải hạng 2 Kimpt Fifa Online 3 Championship - Vòng 2 - Mùa giải 2017/2018 - Duration: 2:05:22.

-------------------------------------------

Japanese Girl Singing In Maid Cosplay Costume (WATCH TILL END - Sing Cuter and Better Near The End) - Duration: 1:54.

Cute Japanese Girl in Maid Cosplay Costume Singing

For more infomation >> Japanese Girl Singing In Maid Cosplay Costume (WATCH TILL END - Sing Cuter and Better Near The End) - Duration: 1:54.

-------------------------------------------

You gotta be kitten me! Rainy Nights Part 22 - Miraculous Ladybug Comic - Duration: 1:16.

For more infomation >> You gotta be kitten me! Rainy Nights Part 22 - Miraculous Ladybug Comic - Duration: 1:16.

-------------------------------------------

Hoe een achterste remtrommel en remblokken vervangen op een FIAT PUNTO HANDLEIDING | AUTODOC - Duration: 6:11.

Use a socket №12

Remove the wheel hub cap

Use a socket №32

Treat the brake drum seat with a copper grease

For more infomation >> Hoe een achterste remtrommel en remblokken vervangen op een FIAT PUNTO HANDLEIDING | AUTODOC - Duration: 6:11.

-------------------------------------------

How to connect a smartphone, tablet or laptop as a network monitor in Windows 10? Spacedesk! - Duration: 4:20.

Hello!

Are you Comp Help channel.

In today's video we to talk about you that

how to connect a laptop, smartphone or tablet to

Windows PCs 10 as a network monitor.

In multi-monitor systems traditionally used

conventional monitors for computers, but instead with the same

can be successfully used smartphones, tablets, laptops

or conventional desktop computers.

But this does not necessarily cables to connect devices.

If your gadgets are connected to the same local network,

will be sufficient in all only one set

instance under the program called Spacedesk.

This small utility is fully is free and allows you to convert

any device running Windows and Android systems in full

Network Monitor.

The program consists of two parts. 1 I have the server-side

installed on your computer from which will go Webcast

monitor, and the second client part of the set

to the mobile device, tablet or other PC.

Let's move on to the site program booted

and installing a server part on computer.

Also a little later from his smartphone I separately upload

the client part.

Link to the website where you can download all the parts of the program

will be given in the description roller.

Downloaded.

Set.

We agree with the conditions.

That program is installed.

Now you are downloading client-side program

in my case a smartphone.

On the site click on the icon that you need I click

on the Android icon and then I slung the Play

Market where I was producing plant client.

Installation completed.

Now I open the app.

After starting my shows this window.

Where the list is my computer, it will be displayed if

it is running the server and part of it is on the same network

with a client I have these conditions respected.

And I'm connected to it connects to.

After connecting with I smartphone can manage

your computer.

I just drive a finger on screen smartphone and the

most run computer mice I think is very convenient,

for example at night when laziness wake up with a cozy crib

to turn off the computer.

In addition, instead of the smartphone or plate, as

second client monitor You can use any

Windows PCs, as mentioned earlier

in the video for broadcast Pictures can be used

browser with support for HTML5, e.g. Google Chrome.

But when using the browser, it is necessary that the device

They were cabled and from such high translation

image quality expected not worth it.

So it is better to establish client on the PC.

He provides more opportunities.

Here the user can configure the extension to duplicate

basic image screen computer on a network display,

stretch your desktop, and select the quality

the displayed image.

To connect to the server part of the program is sufficient

run the client push under the item file and click

on connect icon.

Then you can manage connected computer

from another PC.

I also want to note that Support for Windows 10, this

the program is declared to the version Anniversary Edition, interface language

program unfortunately only English, but looking

Video is going to see tooltip you learn

how to translate the text into any place on your computer.

Also computers running Windows 7 and 8.1 are not

be a server, but only client.

Server can only be computer running

Windows 10, in addition to these restrictions the program also does not support

work with virtual machines and Microsoft Edge browsers and

Mozilla Firefox.

That's basically it.

I hope this program It will be useful to many and

It simplifies your life.

Thank you all for watching.

Thank you that you have 10,000 thousand.

I am very happy and every day I will improve your channel

this figure rose to factor of.

Loved video set Like, who does not put

dislayk, write comments who do not understand, click

Bell not to miss the following video and subscribe

groups in social networks, as well as my Youtube

Telegram and channels all links in description.

Bye everyone and see you in the next video!

For more infomation >> How to connect a smartphone, tablet or laptop as a network monitor in Windows 10? Spacedesk! - Duration: 4:20.

-------------------------------------------

Opel Corsa 1.2-16V EDITION - NAVIGATIE - AIRCO - BLEUTOOTH - RIJKLAAR ! - Duration: 1:01.

For more infomation >> Opel Corsa 1.2-16V EDITION - NAVIGATIE - AIRCO - BLEUTOOTH - RIJKLAAR ! - Duration: 1:01.

-------------------------------------------

Deckenventilator Libelle von CasaFan - creoven.tv Produktvideo - Duration: 1:14.

For more infomation >> Deckenventilator Libelle von CasaFan - creoven.tv Produktvideo - Duration: 1:14.

-------------------------------------------

New 2017 Honda Civic Type R

For more infomation >> New 2017 Honda Civic Type R

-------------------------------------------

Relaxing Flute: Beautiful Flute Melodies To Calm Thoughts & Relax - Duration: 1:02:11.

Relaxing Flute: Beautiful Flute Melodies To Calm Thoughts & Relax

For more infomation >> Relaxing Flute: Beautiful Flute Melodies To Calm Thoughts & Relax - Duration: 1:02:11.

-------------------------------------------

Ask Admissions: What should I do to prepare for a college visit? - Duration: 0:46.

Hi y'all. My name is Chris Wallace. I represent the University of Illinois at the

Chicago satellite office as an admissions counselor. Hey everyone, my

name is Kaci Abolt, and I'm an admissions counselor here in the Urbana office.

Today we're going to be answering some questions about campus visits. Absolutely.

So for starters, "What should you do to prepare for a campus visit?" So the

first step is to sign up for a visit. You can do that on your my Illini account, or

you can check out our website to see all of our different visit options. Next I

would actually suggest that you make a list of everything that you would like

to see on your visit before you happen to come.

For more infomation >> Ask Admissions: What should I do to prepare for a college visit? - Duration: 0:46.

-------------------------------------------

What Should I Be For Halloween? - Duration: 3:49.

we are on our way to the vet hospital because we have a vacation coming up and

the dogs are like behind on their shots well one of their shots I think and they

won't do it without an exam so they're gonna get a full exam and then we're

gonna get some shot and they're really really excited because I think they

think they're going somewhere fun but we're not going to good water yeah well

I don't know we're like kind of I'm gonna go in and tell them that we're

here hi nope okay stop maybe I don't know oh my oh she just

grabbed my leg all right Shh how much was the how much did she weigh huh

so she went down it was like 98 I think or something oh my goodness what are you

doing got the kiddos and we're gonna go with her store shopping Gracie's all

embarrassed about gear room but we need to get stuff for winter

alright so we just finished at the thrift store they don't really have a

great selection right now we were looking for stuff that's like you know

sweaters long-sleeve shirts stuff of that nature I only got one shirt and

Gracie got a pair of boots but that's pretty much it but we had a lot of fun

like looking through the Halloween stuff I was gonna be a vampire but Hannah

wants to be a vampire so I don't want to do the same thing it's not figure out

what else I'm gonna do yeah now we're gonna just head home and have some lunch

and I'm not sure what else we're gonna do today

well now it is Sunday afternoon literally nothing has happened this

whole weekend which on one side of is like super nice like Ted not do anything

for once but not good for vlogging content so I'm not gonna I'm not gonna

try to stretch it out and like fill this video in with just boring stuff I'm

gonna go ahead and probably just end this so it'll be a short vlog but I did

want to let you guys know the next couple vlogs should be pretty fun

because we have family coming into town again so Matt's parents are coming in

next week and I'm so excited we haven't seen them in so long and then Melissa

was just here so we're going on vacation we took five days off so it should be

really really fun and I'm gonna try to like use snapchat a little bit more

while we're there to show you guys like what we're doing sticking if you're

following me there you can kind of see it like more instantly if you're not

following me on snapchat I will leave the little icon right here so you can

follow me I'm gonna be vlogging the whole time obviously but that's pretty

much it for this vlog because like I said we haven't been doing anything and

it's been super boring bye awesome cuz I like not doing stuff sometimes my life

is a little chaotic if you guys like this video please give me a thumbs up

and if you want to see more videos from me then don't forget to subscribe and

hit that little bell so that you get notified when I upload a new video I'll

talk to you later peace

For more infomation >> What Should I Be For Halloween? - Duration: 3:49.

-------------------------------------------

October Breast Cancer Series: What is breast cancer and how can I avoid it? - Duration: 3:52.

For more infomation >> October Breast Cancer Series: What is breast cancer and how can I avoid it? - Duration: 3:52.

-------------------------------------------

Ask Admissions: When should I start visiting colleges? - Duration: 0:28.

"So when should I start visiting colleges?" So we really see students

start visiting at any point in their high school career. You may come with a

high school group as early as your freshman year, but really start thinking

about the schools that you want to visit towards the end to the beginning of your

junior and senior year. Absolutely, I agree.

For more infomation >> Ask Admissions: When should I start visiting colleges? - Duration: 0:28.

-------------------------------------------

Ask Admissions: Does it matter if I go on a campus visit? - Duration: 0:32.

So, our next question is "Does it matter if I go on a campus visit?"

It absolutely matters. You're gonna be spending a lot of money for college.

So, you wanna go and see the colleges that you are actually interested in

prior to choosing to attend. So, yes, it very much so matters if you attend a campus visit.

Most definitely. But it doesn't impact our review process. So, we wouldn't put that into

your application review in any way.

For more infomation >> Ask Admissions: Does it matter if I go on a campus visit? - Duration: 0:32.

-------------------------------------------

Atopic Dermatitis: How can I relieve discomfort when my child has an eczema flare-up? | Mustela - Duration: 2:08.

When a flare-up happens, you need to move on to medication in the form of a cream,

so your doctor has already prescribed or will prescribe a topical corticosteroid.

Topical corticosteroids are completely different from the corticosteroids in tablet form,

commonly known as cortisone.

Topical corticosteroids treat the surface of the skin,

are very effective very quickly and are essential, but only when flare-ups occur.

And topical corticosteroids aren't too dangerous for children,

they can be applied with no problems?

Topical corticosteroid creams do not penetrate into the bloodstream.

I know that is parents' biggest fear, but it doesn't penetrate the blood,

and it doesn't affect the child's immune system, the child will not be infected by applying corticosteroids.

I often hear, 'But doctor, it will thin my child's skin.'

No, if it's properly applied, i.e. if the corticosteroid is applied during the flare-up,

which lasts a week or two, and stopped when the flare-up stops,

it doesn't damage the skin at all, it doesn't thin it.

Very long-term application over months and months might eventually one day thin the skin.

In the case of a flare-up you have to act fast. You have to reduce the inflammation quickly,

and today the only molecule that can do that in the skin is a mild corticosteroid.

That is for rashes, but if you have the time to continue to apply the emollient,

balm or cream to the rest of the skin, which is still dry, remember, that's good too.

So during flare-ups, it's a little corticosteroid on the rash and lots of emollient in the surrounding area.

For more infomation >> Atopic Dermatitis: How can I relieve discomfort when my child has an eczema flare-up? | Mustela - Duration: 2:08.

-------------------------------------------

Honda CR-V 2.0I COMFORT - Duration: 0:59.

For more infomation >> Honda CR-V 2.0I COMFORT - Duration: 0:59.

-------------------------------------------

Hyundai Atos Spirit 1.0i LX - Duration: 0:59.

For more infomation >> Hyundai Atos Spirit 1.0i LX - Duration: 0:59.

-------------------------------------------

Honda HR-V 1.5 i-VTEC ELEGANCE AUTOMAAT - NAVIGATIE - Rijklaar!!! - Duration: 0:56.

For more infomation >> Honda HR-V 1.5 i-VTEC ELEGANCE AUTOMAAT - NAVIGATIE - Rijklaar!!! - Duration: 0:56.

-------------------------------------------

Citroën Xsara Picasso 1.6i-16V Caractère - Duration: 0:50.

For more infomation >> Citroën Xsara Picasso 1.6i-16V Caractère - Duration: 0:50.

-------------------------------------------

Opel Omega 2.0I GL - Duration: 0:54.

For more infomation >> Opel Omega 2.0I GL - Duration: 0:54.

-------------------------------------------

Hyundai Getz 1.1i GL 3DRS - Duration: 0:54.

For more infomation >> Hyundai Getz 1.1i GL 3DRS - Duration: 0:54.

-------------------------------------------

Toyota Corolla Wagon 1.4 VVT-i Linea Terra - Duration: 1:00.

For more infomation >> Toyota Corolla Wagon 1.4 VVT-i Linea Terra - Duration: 1:00.

-------------------------------------------

Hyundai Tucson 2.0i Style Cruise Rijklaar! - Duration: 0:59.

For more infomation >> Hyundai Tucson 2.0i Style Cruise Rijklaar! - Duration: 0:59.

-------------------------------------------

Citroën Berlingo 1.8i Multispace, schuifdeur, trekhaak, nette auto! - Duration: 0:59.

For more infomation >> Citroën Berlingo 1.8i Multispace, schuifdeur, trekhaak, nette auto! - Duration: 0:59.

-------------------------------------------

Toyota iQ 1.0 VVT-i Aspiration Zeer luxe uitv.! - Duration: 0:58.

For more infomation >> Toyota iQ 1.0 VVT-i Aspiration Zeer luxe uitv.! - Duration: 0:58.

-------------------------------------------

Hyundai Tucson 2.0I DYNAMIC - Duration: 0:59.

For more infomation >> Hyundai Tucson 2.0I DYNAMIC - Duration: 0:59.

-------------------------------------------

Opel Corsa 1.2I-16V STRADA 5-DEURS AUTOMAAT - Duration: 0:42.

For more infomation >> Opel Corsa 1.2I-16V STRADA 5-DEURS AUTOMAAT - Duration: 0:42.

-------------------------------------------

Honda HR-V 1.5 i-Vtec Elegance CVT Automaat Rijklaar - Duration: 0:54.

For more infomation >> Honda HR-V 1.5 i-Vtec Elegance CVT Automaat Rijklaar - Duration: 0:54.

-------------------------------------------

New: Osmo MindRacers

For more infomation >> New: Osmo MindRacers

-------------------------------------------

Manhã Leve | Fisioterapeuta ajuda o sr. Luiz a realizar o sonho da filha - - Duration: 22:07.

For more infomation >> Manhã Leve | Fisioterapeuta ajuda o sr. Luiz a realizar o sonho da filha - - Duration: 22:07.

-------------------------------------------

Manhã Leve | Saiba como funciona o esquema de segurança no Santuário Nacional - - Duration: 5:09.

For more infomation >> Manhã Leve | Saiba como funciona o esquema de segurança no Santuário Nacional - - Duration: 5:09.

-------------------------------------------

O QUE O Sr(a) ACHOU DO MEU TRABALHO? - Duration: 1:00.

For more infomation >> O QUE O Sr(a) ACHOU DO MEU TRABALHO? - Duration: 1:00.

-------------------------------------------

Kobiecy kącik: Co myślę o KASIE WEST? [Jestem na TAK] - Duration: 13:59.

For more infomation >> Kobiecy kącik: Co myślę o KASIE WEST? [Jestem na TAK] - Duration: 13:59.

-------------------------------------------

Benjamins - Anseios (Vídeo Oficial) - Duration: 4:57.

For more infomation >> Benjamins - Anseios (Vídeo Oficial) - Duration: 4:57.

-------------------------------------------

Por que a agricultura está com problemas (e como resolver)? | Minuto da Terra - Duration: 3:04.

For more infomation >> Por que a agricultura está com problemas (e como resolver)? | Minuto da Terra - Duration: 3:04.

-------------------------------------------

Bingo Dog Song Kids Songs & Nursery Rhymes Little Baby Playing on the Playground for Children - Duration: 2:07.

Bingo Dog Song Kids Songs & Nursery Rhymes Little Baby Playing on the Playground for Children

For more infomation >> Bingo Dog Song Kids Songs & Nursery Rhymes Little Baby Playing on the Playground for Children - Duration: 2:07.

-------------------------------------------

¿Cómo hacer adornos de navidad en papel? - Duration: 2:25.

For more infomation >> ¿Cómo hacer adornos de navidad en papel? - Duration: 2:25.

-------------------------------------------

Manhã Leve | Aprenda a fazer massinha de modelar - 04 de outubro de 2017 - Duration: 16:30.

For more infomation >> Manhã Leve | Aprenda a fazer massinha de modelar - 04 de outubro de 2017 - Duration: 16:30.

-------------------------------------------

Cuentos Extraños para Niños Peculiares - Duration: 3:37.

For more infomation >> Cuentos Extraños para Niños Peculiares - Duration: 3:37.

-------------------------------------------

Edição Especial Caroline FC(Os Melhores Lances do FIFA no xbox 360) - Duration: 1:46.

The best throws of the FIFA in Xbox 360. Presents !

Caroline FC special edition

Thank you for watching,sign up for the channel.

For more infomation >> Edição Especial Caroline FC(Os Melhores Lances do FIFA no xbox 360) - Duration: 1:46.

-------------------------------------------

[VIDEO 2 de 3] - Curso de Oratoria y de Cómo Hablar en Público | TecnicasHablarEnPublico.com - Duration: 15:01.

For more infomation >> [VIDEO 2 de 3] - Curso de Oratoria y de Cómo Hablar en Público | TecnicasHablarEnPublico.com - Duration: 15:01.

-------------------------------------------

Tutorial Salve o progresso dos seus jogos sem Memory Card no PS2 - Duration: 12:47.

For more infomation >> Tutorial Salve o progresso dos seus jogos sem Memory Card no PS2 - Duration: 12:47.

-------------------------------------------

CONCURSO MAQUINATE! 2 | TU Y YO 😍 - Duration: 1:31.

For more infomation >> CONCURSO MAQUINATE! 2 | TU Y YO 😍 - Duration: 1:31.

-------------------------------------------

Biggest Deal TVI - Os 'podres' de Eduardo Beauté revelados - Duration: 2:05.

For more infomation >> Biggest Deal TVI - Os 'podres' de Eduardo Beauté revelados - Duration: 2:05.

-------------------------------------------

How to connect a smartphone, tablet or laptop as a network monitor in Windows 10? Spacedesk! - Duration: 4:20.

Hello!

Are you Comp Help channel.

In today's video we to talk about you that

how to connect a laptop, smartphone or tablet to

Windows PCs 10 as a network monitor.

In multi-monitor systems traditionally used

conventional monitors for computers, but instead with the same

can be successfully used smartphones, tablets, laptops

or conventional desktop computers.

But this does not necessarily cables to connect devices.

If your gadgets are connected to the same local network,

will be sufficient in all only one set

instance under the program called Spacedesk.

This small utility is fully is free and allows you to convert

any device running Windows and Android systems in full

Network Monitor.

The program consists of two parts. 1 I have the server-side

installed on your computer from which will go Webcast

monitor, and the second client part of the set

to the mobile device, tablet or other PC.

Let's move on to the site program booted

and installing a server part on computer.

Also a little later from his smartphone I separately upload

the client part.

Link to the website where you can download all the parts of the program

will be given in the description roller.

Downloaded.

Set.

We agree with the conditions.

That program is installed.

Now you are downloading client-side program

in my case a smartphone.

On the site click on the icon that you need I click

on the Android icon and then I slung the Play

Market where I was producing plant client.

Installation completed.

Now I open the app.

After starting my shows this window.

Where the list is my computer, it will be displayed if

it is running the server and part of it is on the same network

with a client I have these conditions respected.

And I'm connected to it connects to.

After connecting with I smartphone can manage

your computer.

I just drive a finger on screen smartphone and the

most run computer mice I think is very convenient,

for example at night when laziness wake up with a cozy crib

to turn off the computer.

In addition, instead of the smartphone or plate, as

second client monitor You can use any

Windows PCs, as mentioned earlier

in the video for broadcast Pictures can be used

browser with support for HTML5, e.g. Google Chrome.

But when using the browser, it is necessary that the device

They were cabled and from such high translation

image quality expected not worth it.

So it is better to establish client on the PC.

He provides more opportunities.

Here the user can configure the extension to duplicate

basic image screen computer on a network display,

stretch your desktop, and select the quality

the displayed image.

To connect to the server part of the program is sufficient

run the client push under the item file and click

on connect icon.

Then you can manage connected computer

from another PC.

I also want to note that Support for Windows 10, this

the program is declared to the version Anniversary Edition, interface language

program unfortunately only English, but looking

Video is going to see tooltip you learn

how to translate the text into any place on your computer.

Also computers running Windows 7 and 8.1 are not

be a server, but only client.

Server can only be computer running

Windows 10, in addition to these restrictions the program also does not support

work with virtual machines and Microsoft Edge browsers and

Mozilla Firefox.

That's basically it.

I hope this program It will be useful to many and

It simplifies your life.

Thank you all for watching.

Thank you that you have 10,000 thousand.

I am very happy and every day I will improve your channel

this figure rose to factor of.

Loved video set Like, who does not put

dislayk, write comments who do not understand, click

Bell not to miss the following video and subscribe

groups in social networks, as well as my Youtube

Telegram and channels all links in description.

Bye everyone and see you in the next video!

For more infomation >> How to connect a smartphone, tablet or laptop as a network monitor in Windows 10? Spacedesk! - Duration: 4:20.

-------------------------------------------

Pesca de Tilápia na Vara de Mão - Paraíso das Tilaponas - Duration: 2:34.

For more infomation >> Pesca de Tilápia na Vara de Mão - Paraíso das Tilaponas - Duration: 2:34.

-------------------------------------------

Peppa Pig George m&m Chocolates Finger Family Song Colors Learn - Duration: 1:32.

Peppa Pig George m&m Chocolates Finger Family Song Colors Learn