Youtube daily report w Jul 22 2017

Collinearity, Betweenness, and Assumptions, level 4.

In this final video we will go over 4 examples that involve angle measurements.

We will be making assumptions from diagrams in order to solve these problems.

Let's start with the first example.

Find the measure of angle ABC.

In this problem we are provided with a diagram and are asked to find the measurement of angle

ABC.

We can determine the measurement of this angle by finding the value of the variable X and

then we substitute this value into the algebraic expression of the angle and simplify.

We first need to set up a geometric relation that will allow us to solve for the variable

X. Notice that points A, B and D are collinear this means that they lie in the same line.

This also means that this line represents a straight angle.

Recall that straight angles measure 180 degrees.

Also notice that this straight angle is formed by two angles in this case angle ABC and angle

CBD.

So the sum of the measures of angle ABC plus angle CBD must be equal to 180 degree since

both angles form a straight angle.

We can now substitute the algebraic expressions for each angle into this geometric relation

doing that we obtain the following equation.

Now it is just a matter of simplifying and collecting like terms.

Solving for the unknown variable we obtain x equals 42.

We now have to substitute this value into the algebraic expression representing the

measurement of angle ABC doing that and simplifying we obtain 134 degrees and this is our final

answer.

Let's try the next example.

Angle ABC is a right angle.

The ratio of the measures of angle ABD and angle DBC is 3 to 2.

Find the measure of angle ABD.

In this problem we are provided with a diagram of a right angle that is formed by two smaller

angles.

We are given a ratio between the two smaller angles and are asked to determine the measurement

of angle ABD.

In order to determine the measurement of angle ABD we need to figure out the combination

of angles that would add up to 90 degrees and have a ratio of 3 to 2.

In other words the measurement of angle ABD plus the measurement of angle DBC should equal

the measurement of angle ABC which is a right angle so the measurements should add to 90

degrees.

Also the ratio between the measurement of angle ABD and the measurement of angle DBC

should equal 3 over 2.

We can systematically go through various angles and determine what combination of angles meets

these two constraints or we can solve the problem by using algebra.

We can write an equivalent ratio by multiplying the fraction 3 over 2 by x over x.

This way we can now replace the measure of angle ABD with 3x and replace the measure

of angle DBC with 2x.

We then use these algebraic expressions and substitute them into the geometric relation,

doing that we obtain the following equation.

Now it is just a matter of simplifying the equation and solving for x, doing that we

obtain x equals 18.

We then use this value of x to find the measure of angle ABD doing that we obtain 54 degrees

and this is our final answer.

Let's move along to the next example.

The measure of angle 1, angle 2 and angle 3 are in the ratio 1 to 3 to 2.

Find the measure of each angle.

Similar to the previous problem we are provided with a diagram with various angles.

We are given the ratio of the measurement between all 3 angles.

We are asked to find the measurement of all three angles.

Let's first determine the geometric relation of the problem.

Notice that angle 1, angle 2 and angle 3 form a straight angle.

So we can set up the following geometric relation, the measure of angle 1 plus the measure of

angle 2 plus the measure of angle 3 is equal to 180 degree.

Next we need to determine the algebraic relations between the angles, since we know that the

ratio between the measures of the angles is 1 to 3 to 2 if we multiply all the values

of this ratio by x we should obtain an equivalent ratio.

By doing this we can now assign an algebraic expression to each of the three angles.

We can now use these algebraic expressions and substitute them into the geometric relation

doing that we obtain the following.

Now it is just a matter of simplifying the expression and solving for X, doing that we

obtain X equals 30.

The last step is to substitute this value of X into the algebraic expression of each

angle doing that we obtain 30, 90 and 60 degrees and this is our final answer.

Alright let's end the video by going over the final example.

Given the measure of angle 1 equals 2x plus 40, the measure of angle 2 equals 2y plus

40, the measure of angle 3 equals x plus 2y, find the measure of angle 1, the measure of

angle 2 and the measure of angle 3.

In this problem we are provided with a diagram and are given algebraic relations for 3 angles.

We are asked to determine the measurement of all 3 angles.

For this problem we are first going to determine the geometric relations between the various

angles.

Notice that angle 1 and angle 2 form a straight angle so we can set up the following geometric

relation the measurement of angle 1 plus the measurement of angle 2 is equal to 180.

In a similar fashion angle 2 and angle 3 also form a straight angle so we can set up the

following geometric relation the measurement of angle 2 plus the measurement of angle 3

equals 180 degrees.

Now that we have these two geometric relations let's go ahead and substitute the measurement

of the angles with the given algebraic expressions.

Doing that we obtain the following.

Let's go ahead and simplify both of these equations by collecting like terms and keeping

the variables on the left side of the equation and any constants on the right side of the

equation doing that we obtain the following.

We are now face to face with a system of linear equations so we will use the substitution

method to solve this system.

Solving for x in the first equation we obtain the following.

Now we go ahead and substitute this expression into the second equation.

From here it is just a matter of simplifying and collecting like terms, we then solve for

the variable y doing that we obtain y equals 30.

Next we take this value for y and substitute it into the first equation this allows us

to solve for the variable x doing that we obtain x equals 20.

The last step is to evaluate the algebraic expression for each of the 3 angles we do

this by substituting the values for x and y.

Doing that and simplifying we obtain 80 degrees for the measurement of angle 1, 100 degrees

for the measurement of angle 2 and 80 degrees for the measurement of angle 3 and this is

our final answer.

In our next series of videos we will start learning how to write simple proofs.

For more infomation >> Geometry: Collinearity, Betweenness, and Assumptions (Level 4 of 4) | Examples III - Duration: 8:31.

-------------------------------------------

Former Buc Owenby and Danville get past Cards; Bluefield outlast E-Twins - Duration: 1:29.

For more infomation >> Former Buc Owenby and Danville get past Cards; Bluefield outlast E-Twins - Duration: 1:29.

-------------------------------------------

Análise e Palpite Avai x Cruzeiro Rodada 16 | Prognósticos de Futebol Brasileirão 2017 - Duration: 2:53.

For more infomation >> Análise e Palpite Avai x Cruzeiro Rodada 16 | Prognósticos de Futebol Brasileirão 2017 - Duration: 2:53.

-------------------------------------------

Kiev Ballet - Olá Brasil - Kateryna Kozachenko - Duration: 4:31.

For more infomation >> Kiev Ballet - Olá Brasil - Kateryna Kozachenko - Duration: 4:31.

-------------------------------------------

五月天阿信「四字告白」！被機車亂入搞砸　谷歌大神幫標記 - Duration: 2:14.

For more infomation >> 五月天阿信「四字告白」！被機車亂入搞砸　谷歌大神幫標記 - Duration: 2:14.

-------------------------------------------

Geometry: Collinearity, Betweenness, and Assumptions (Level 4 of 4) | Examples III - Duration: 8:31.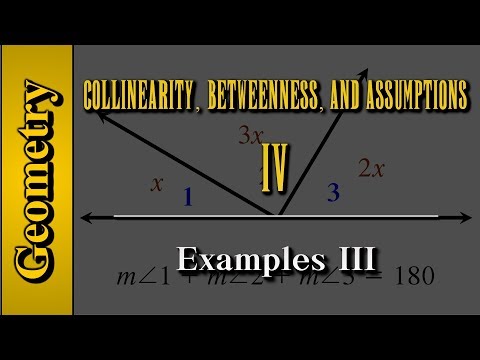

Collinearity, Betweenness, and Assumptions, level 4.

In this final video we will go over 4 examples that involve angle measurements.

We will be making assumptions from diagrams in order to solve these problems.

Let's start with the first example.

Find the measure of angle ABC.

In this problem we are provided with a diagram and are asked to find the measurement of angle

ABC.

We can determine the measurement of this angle by finding the value of the variable X and

then we substitute this value into the algebraic expression of the angle and simplify.

We first need to set up a geometric relation that will allow us to solve for the variable

X. Notice that points A, B and D are collinear this means that they lie in the same line.

This also means that this line represents a straight angle.

Recall that straight angles measure 180 degrees.

Also notice that this straight angle is formed by two angles in this case angle ABC and angle

CBD.

So the sum of the measures of angle ABC plus angle CBD must be equal to 180 degree since

both angles form a straight angle.

We can now substitute the algebraic expressions for each angle into this geometric relation

doing that we obtain the following equation.

Now it is just a matter of simplifying and collecting like terms.

Solving for the unknown variable we obtain x equals 42.

We now have to substitute this value into the algebraic expression representing the

measurement of angle ABC doing that and simplifying we obtain 134 degrees and this is our final

answer.

Let's try the next example.

Angle ABC is a right angle.

The ratio of the measures of angle ABD and angle DBC is 3 to 2.

Find the measure of angle ABD.

In this problem we are provided with a diagram of a right angle that is formed by two smaller

angles.

We are given a ratio between the two smaller angles and are asked to determine the measurement

of angle ABD.

In order to determine the measurement of angle ABD we need to figure out the combination

of angles that would add up to 90 degrees and have a ratio of 3 to 2.

In other words the measurement of angle ABD plus the measurement of angle DBC should equal

the measurement of angle ABC which is a right angle so the measurements should add to 90

degrees.

Also the ratio between the measurement of angle ABD and the measurement of angle DBC

should equal 3 over 2.

We can systematically go through various angles and determine what combination of angles meets

these two constraints or we can solve the problem by using algebra.

We can write an equivalent ratio by multiplying the fraction 3 over 2 by x over x.

This way we can now replace the measure of angle ABD with 3x and replace the measure

of angle DBC with 2x.

We then use these algebraic expressions and substitute them into the geometric relation,

doing that we obtain the following equation.

Now it is just a matter of simplifying the equation and solving for x, doing that we

obtain x equals 18.

We then use this value of x to find the measure of angle ABD doing that we obtain 54 degrees

and this is our final answer.

Let's move along to the next example.

The measure of angle 1, angle 2 and angle 3 are in the ratio 1 to 3 to 2.

Find the measure of each angle.

Similar to the previous problem we are provided with a diagram with various angles.

We are given the ratio of the measurement between all 3 angles.

We are asked to find the measurement of all three angles.

Let's first determine the geometric relation of the problem.

Notice that angle 1, angle 2 and angle 3 form a straight angle.

So we can set up the following geometric relation, the measure of angle 1 plus the measure of

angle 2 plus the measure of angle 3 is equal to 180 degree.

Next we need to determine the algebraic relations between the angles, since we know that the

ratio between the measures of the angles is 1 to 3 to 2 if we multiply all the values

of this ratio by x we should obtain an equivalent ratio.

By doing this we can now assign an algebraic expression to each of the three angles.

We can now use these algebraic expressions and substitute them into the geometric relation

doing that we obtain the following.

Now it is just a matter of simplifying the expression and solving for X, doing that we

obtain X equals 30.

The last step is to substitute this value of X into the algebraic expression of each

angle doing that we obtain 30, 90 and 60 degrees and this is our final answer.

Alright let's end the video by going over the final example.

Given the measure of angle 1 equals 2x plus 40, the measure of angle 2 equals 2y plus

40, the measure of angle 3 equals x plus 2y, find the measure of angle 1, the measure of

angle 2 and the measure of angle 3.

In this problem we are provided with a diagram and are given algebraic relations for 3 angles.

We are asked to determine the measurement of all 3 angles.

For this problem we are first going to determine the geometric relations between the various

angles.

Notice that angle 1 and angle 2 form a straight angle so we can set up the following geometric

relation the measurement of angle 1 plus the measurement of angle 2 is equal to 180.

In a similar fashion angle 2 and angle 3 also form a straight angle so we can set up the

following geometric relation the measurement of angle 2 plus the measurement of angle 3

equals 180 degrees.

Now that we have these two geometric relations let's go ahead and substitute the measurement

of the angles with the given algebraic expressions.

Doing that we obtain the following.

Let's go ahead and simplify both of these equations by collecting like terms and keeping

the variables on the left side of the equation and any constants on the right side of the

equation doing that we obtain the following.

We are now face to face with a system of linear equations so we will use the substitution

method to solve this system.

Solving for x in the first equation we obtain the following.

Now we go ahead and substitute this expression into the second equation.

From here it is just a matter of simplifying and collecting like terms, we then solve for

the variable y doing that we obtain y equals 30.

Next we take this value for y and substitute it into the first equation this allows us

to solve for the variable x doing that we obtain x equals 20.

The last step is to evaluate the algebraic expression for each of the 3 angles we do

this by substituting the values for x and y.

Doing that and simplifying we obtain 80 degrees for the measurement of angle 1, 100 degrees

for the measurement of angle 2 and 80 degrees for the measurement of angle 3 and this is

our final answer.

In our next series of videos we will start learning how to write simple proofs.

For more infomation >> Geometry: Collinearity, Betweenness, and Assumptions (Level 4 of 4) | Examples III - Duration: 8:31.

-------------------------------------------

Big Brother - Cody And Paul Go Head To Head During The Big Brother Battle Back Showdown - Duration: 5:09.

>> I KNEW I'D BEAT CODY IN HIS OWN GAME A FEW WEEKS AGO AND

SENT HIM BACK IN THE HOUSE BUT IF HE COMES BACK TO THE HOUSE IT

WILL BE A WHOLE OTHER HEADACHE AND HE'LL BE GUNNING FOR ME SO I

HAVE TO PULL THIS OUT AND MAKE SURE HE DOESN'T COME BACK IN.

>> NOW THAT I'VE MADE IT THIS FOR I CAN'T LOSE.

JESS IS LOOKING ON.

EVERYONE'S LOOKING ON.

IT'S THE BAT OF THE ALPHAS.

WE HAVE FACED OFF BEFORE AND I CAME OUT ON TOP SO I HAVE TO WIN

THIS THING TO BET BACK IN THE HOUSE.

>> THIS COMPETITION STARTS NOW.

>> CODY'S PLAYED THIS BEFORE AND I'M PULLING ONE WAY AND IT'S

GOING THE OTHER WAY.

NOT AS EASY AS A THOUGHT.

>> THOUGH I'VE DONE THIS BEFORE IT'S A DIFFERENT MAZE SO I HAVE

TO MAINTAIN MY FOCUS.

THIS IS ANYBODY'S GAME.

COME ON, PAUL.

YOU NEED TO WIN THIS SHOWDOWN AND SEND CODY HOME FOR GOOD THIS

TIME.

>> I AM PRETTY SURE NONE OF THEM WANT CODY BACK IN THE HOUSE SO I

HAVE TO PULL THIS OUT, PAUL.

COME ON, PAUL, DO IT FOR FRIENDSHIP.

DO IT FOR THE HOUSE GUEST.

KEEP CODY OUT OF THE GAME.

>> I GLANCE OVER AND LOOK AT PAUL'S MAZE.

HE'S BEHIND ME.

I HAVE TO MAINTAIN A SLOW AND STEADY PACE AT THIS POINT.

>> THE LONGER I PLAY THIS GAME THE MORE I GET THE FEEL FOR THIS

THING AND SLOWLY START TO PICK UP THE PACE.

I DON'T WANT TO PAY ATTENTION TO CODY'S BOARD AND FREAK OUT.

I'M CONCENTRATING ON MY BALL AND MAKING SURE IT'S NOT GOING

ANYWHERE NEAR ANY HOLE.

>> I HIT PANIC MODE WHEN CODY GETS IN THE LEAD.

AT THIS POINT I'M LOSING IT.

I'M THINKING HE HAS A GOOD LEAD ON PAUL AND THIS KID MIGHT BE

WALKING BACK IN THE HOUSE.

>> PAUL'S FINE BUT WE JUST NEED CODY'S BALL TO DROP AND IT COULD

BE A GAME CHANGER.

WE CAN PULL AHEAD AT ANY MOMENT.

>> CODY'S IN THE LEAD AND IF HE BLOWS THIS I WILL KILL HIM.

I NEED HIM IN THE GAME.

I CAN'T DO IT ALONE.

PLEASE, CODY, WIN THIS FOR ME.

>> CONGRATULATIONS, CODY, YOU HAVE WON THE BATTLE BACK

SHOWDOWN AND YOU ARE BACK IN THE GAME.

>> THIS IS DIVINE INTERVENTION.

I'M HAPPY I WON THIS.

I GET TO SPEND MORE TIME WITH JESS AND PAUL SHOULD HAVE BET ON

THE BATTLE BACK WHICH HE DIDN'T.

NOW WHEN I'M BACK IN THE GAME HIS SAFETY IS OVER.

ONCE I GET THE CHANCE I'M GOING TO TAKE HIM OUT.

>> CODY FINISHES THE MAZE AND NOW HE'S BACK IN THE HOUSE.

IT'S THE BEST CASE SCENARIO.

I'M SO HAPPY.

I'VE BEEN PLAYING THIS GAME ALLE

ALLE ALLEN -- ALONE AND BETWEEN HIM

AND I WE CAN TURN THE GAME AROUND.

For more infomation >> Big Brother - Cody And Paul Go Head To Head During The Big Brother Battle Back Showdown - Duration: 5:09.

-------------------------------------------

Ravi from VIXX is thrilled to see you at VIXX FAN MEETING TOUR IN SE-ASIA! - Duration: 0:20.

Hey Starlights in Southeast Asia!

This is Ravi from VIXX. Nice to meet you~

It's already getting so close to meet you

I am looking forward to it already

I hope you guys are excited as well

and have a great time with us

See you soon~

For more infomation >> Ravi from VIXX is thrilled to see you at VIXX FAN MEETING TOUR IN SE-ASIA! - Duration: 0:20.

-------------------------------------------

Fuel For The Real Fit - Play School Teacher Story - Duration: 2:49.

Fuel For The Real Fit - Play School Teacher Story

Fuel For The Real Fit - Play School Teacher Story

Fuel For The Real Fit - Play School Teacher Story

For more infomation >> Fuel For The Real Fit - Play School Teacher Story - Duration: 2:49.

-------------------------------------------

Austin experiencing labor shortage amid booming housing market - Duration: 2:39.

For more infomation >> Austin experiencing labor shortage amid booming housing market - Duration: 2:39.

-------------------------------------------

Careers and jobs in fine arts - Duration: 7:46.

WHATS UP my dudes what's going on what's cracking well we bout to get it crackin

today fresh out of the shower I got a little bit of water on the lens so guess

what I need my coffee I'm just fresh out the shower and i don't got

my coffee then would I need my coffee guess what I got a new mug breath

Wingardium Leviosa wassup bruh Gryffindor Harry Potter abracadabra all

them haters not me Voldemorte on the beat let's get my conference because I don't

have a coffee really know how this is this stuff goes down rain got my coffee

know how crazy this is fresh out the shower it'll fresh out the "ligo" shout

out to my subscribers up in the Philippines kumusta kayo! shout out to my

subscribers out there in Spanish countries como estas shout out to all my

Japanese subscribers I don't know how to say yes well yeah shout out to everyone

shout out to the world what's going on you guys subscribe to hit that subscribe

about to do this right now get it go get it start the show welcome back to

the show today we're doing something a little bit different today we're going

to be going over three jobs or three careers that I would like to do and what

we're doing we're going to do first one dancer second rapper and third we're

going to be doing musician so first let's take a look insomniac events it's

pretty much common knowledge that dancing sits at the core of every event

what exactly is the requirements for me to be a dancer let's check this out I

want to be a go-go dancer let's check this out so it says if all I got to do

is wear fitted clothing crop top shorts for females heels wedges or boots

performance hair got that makeup got that

please bring non-returnable dance resume and headshot

alright refillable water bottle alright valid form ID all right cool

what if I was a dancer

i'm not young anymore how do people dance like this

I can't even breathe right now what else can i be.. a dancer or a go-go dancer I can't breathe

i'm about to have an asthma attack

..help

okay maybe I'm just dreaming a little too much what about what if I was just a

Glover

you

all right so I don't know maybe just dancing isn't for me

yeah let's take a look at the next one a rapper let's see what it takes to be a

rapper let's do this degree level none wahoo I Hank got no degree so we good I

could be a rapper was good what's up Gavin on them let's do this

experience training intense music rehearsal practice and performance I got

that I can do this let's see key skills talent discipline

determination I got that stamina I got that some confidence oh shoot I ain't

got no son confidence aw crap crap can be a frigging rapper crap can't be a

frickin rapper good people skills I can't be a rapper

I ain't got no people skills marketing I can market though salary about 24 24

hours I can do this let's try it well if I was a rapper

abstract discovered, i'm chillin on the Youtube. They be like sub for sub dude.

I'm all about that watch time, I don't give a damn about the views dude. ADHD generation at

the beginning of the vlog! My YouTube dream got lost in the fog now I'm out

here trying to abstract discover a job. Is it time to leave? I have no escape

plan and I just got a few subscribers from Japan, I can't let them down

no sayonara! it's loyalty over royalty but let the king take a

seat and pass me the tiarahhhhh! pass me the tiara okay okay maybe I'm dreaming a

little too much in this video let's try the next one

musician alright what's it take to be a musician let's check median pay twenty

four twenty per hour alright twenty four twenty not that much

remember on my old video the brand ambassador they make about

the same, entry-level. No formal education! whoa! all these jobs got no

education required! yeah I got no education! Got a High school diploma though.

Projected ten-year grow three percent number of jobs 173,000 okay what if I was a

musician

okay so my my career job hunting has ended for today's I'm gonna be doing

these skits every Friday so be sure to hit that

subscribe button if you want more because if you want more you gotta hit

that subscribe button it's not I don't know what's wrong with you hit that

subscribe button if you want to see more of these videos and will come back

tomorrow and we'll return to our regular scheduled program and remember these are

every Friday so hit that subscribe a right now so you can not miss out you

don't want to miss out why would you want to miss out tell myself let's do

this woo woo abracadabra on them haters boo boo

Peace Love God bless connect again soon

For more infomation >> Careers and jobs in fine arts - Duration: 7:46.

-------------------------------------------

Superhero And Scream Nerf Gun vs Zombies / Kid Spiderman & Spiderman kill Boss Zombie in the City - Duration: 14:32.

For more infomation >> Superhero And Scream Nerf Gun vs Zombies / Kid Spiderman & Spiderman kill Boss Zombie in the City - Duration: 14:32.

-------------------------------------------

Dinosaur Walking and Laying Eggs Toy - Bad Dinosaur Steals Eggs Mommy to the Rescue - NomNom TOYS - Duration: 3:56.

NomNom TOYS

Dinosaur Walking and Laying Eggs Toy - Bad Dinosaur Steals Eggs - Mommy to the Rescue

A fun dinosaur toy video for kids

For more infomation >> Dinosaur Walking and Laying Eggs Toy - Bad Dinosaur Steals Eggs Mommy to the Rescue - NomNom TOYS - Duration: 3:56.

-------------------------------------------

Просыпайся продуктивным! - Duration: 5:48.

For more infomation >> Просыпайся продуктивным! - Duration: 5:48.

-------------------------------------------

XSMB 22/7 | Phân tích chi tiết XSMB hôm nay 22-7-2017 | Chốt số giờ vàng SXMB Thứ 7 - Duration: 6:10.

For more infomation >> XSMB 22/7 | Phân tích chi tiết XSMB hôm nay 22-7-2017 | Chốt số giờ vàng SXMB Thứ 7 - Duration: 6:10.

-------------------------------------------

XSMB | Dự đoán xổ số Miền Bắc hôm nay 22/7/2017 | BTL xổ số MB thứ 7 22-7-2017 - Duration: 3:52.

For more infomation >> XSMB | Dự đoán xổ số Miền Bắc hôm nay 22/7/2017 | BTL xổ số MB thứ 7 22-7-2017 - Duration: 3:52.

-------------------------------------------

DISHONORABLY LOW BUDGET POWER RANGERS TRAILER!! - Duration: 3:43.

None of us really know eachother...We're ALL screw ups!

But somehow...we were all at the same place at the same time when Billy found those coins!

"Whooooaaaaaaa!!!" *splash* Check out how we glow!

I'm Blue!

Wow, I'm black!

What?

No you're not! *bad giggling* Do you feel weird?

We're strong...INSANELY strong!

The answer to what is happening to you is here: you five are the power rangers.

Where were you last night?

Me and four kids found a spaceship last night...Pretty sure I'm a super hero.

WHOA COOL!

Pee in that cup!

The Power Rangers are a legion of warriors...you must become those warriors.

Man what is this, some kind of simulation?

MAN I need a hotdog! *pants* Holy..GOD...that sucked.

What kind of simulation?

What is this, Watson from IBM?

Call my mom!

MOM!!

AGGHH!!

Mom, I'm not a Power Ranger anymore! *cries* *kissing sounds* *music throughout* She is

PURE Evil...*Evil cackling* It's MORPHIN' time!

"No one man should have all this power..." *fighting sounds* It's MOPHIN' time!

Mastodon!

Pterdactly ! Triceratops!

Saber-tooth tiger!

Tyrannosaurus!

*Power Rangers theme plays* POWER RANGERS!!

For more infomation >> DISHONORABLY LOW BUDGET POWER RANGERS TRAILER!! - Duration: 3:43.

-------------------------------------------

Distracted driving law washington - Duration: 2:09.

Washington's newest distracted-driving law, which aims to curb unsafe driving due to gadget use behind the wheel, goes into effect Sunday. Here's what's changing:

"The big change is you can no longer hold an electronic device — whether it's an iPad, phone or what — while you're operating your vehicle," Clark County sheriff's Detective Todd Young said. "This also includes when stopped in your vehicle."

Currently, the law doesn't necessarily preclude sending texts while stopped at a traffic light. The law is older than the newest technology and was aimed at phones being held to the ear.

The new law, signed by Gov. Jay Inslee on May 16, was broadened to describe more toys and behaviors that aren't allowed behind the wheel

It includes typing messages, accessing information, watching videos or using cameras, according to the Washington Traffic Safety Commission.

Drivers may still use their phone if they're contacting emergency services. Or, if they're parked out of the flow of traffic or starting up music or a GPS system before a trip.

Two-way radios, citizens band radio or amateur radio equipment are not part of the law's scope. Hands-free use through a wireless transmitter — Bluetooth, for example — is OK. Drivers can also use their device if it's hands-free and can be started using a single touch, such as a GPS device.

Transit and emergency vehicle drivers are also exempt, and commercial vehicle drivers are still bound by federal law. Fiddling with the phone while it's in your lap or sitting in the cup holder doesn't count,

Young said: "Obviously, in your lap, you're not going to be looking at the roadway," he said.

Negotiations on that bill collapsed, and the Legislature adjourned without taking up either bill, though lawmakers say they will continue negotiations in hopes of being able to return to the Capitol for a one-day session for votes at a later date.The Associated Press contributed to this report.

For more infomation >> Distracted driving law washington - Duration: 2:09.

-------------------------------------------

龙珠超【更99集】2017 - Duration: 19:26.

For more infomation >> 龙珠超【更99集】2017 - Duration: 19:26.

-------------------------------------------

Gevalia House Blend Coffee Review - Duration: 3:45.

Welcome to Coffee Coffee Coffee, your place for average Joe coffee reviews

I'm J.R. And I'm Kayla. And Kayla what are we here to do today? We're gonna

review Gevalia Caffe House Blend. It's a medium roast. 100% Arabica. Supposed to be

a medium bodied smooth coffee. It also says on the back it's a rich never

bitter experience and it should have, according to them some caramel notes and

a citrus finish. Ah a caramel covered lime. No not that. No so let us see now we

have we have tasted it before but as we've said in other videos

we like to drink before also so that way we can really taste the whole cup as

we go through. Yeah so one thing.. Sorry. I actually did kind

of smell a little bit of like chocolate. A little smell of it. That's not what

this bag says. No. They mixed up their bags.

So a little bit more about Gevalia. It's a Swedish company founded in 1853.

Borshka Borshka. Bork. Bork. Yeah Swedish Chef. Ah, but this is a part of

their heritage blend which is supposed to just be like a better version of your

everyday coffee. Or it's old beans.

We got this from the Heritage Collection. That's in the basement next to all the old shoes. Okay. Dutch

factory. All right so what were your thoughts on the coffee? I have my thoughts

but I want to know your thoughts. Actually the drink I just took I

thought that's fine. Yeah I like it. We we had not this same blend over at

your mom's and so it's been a while since we've had Gevalia and the first

thing I noticed about it was it was a little bit

bitter but that's not necessarily a bad thing I mean bitterness is okay it's you

know not supposed to be really sweet. Um but it's not overpowering. Right.

I felt like um it had almost like a nutty flavor a little bit of the like

the chocolaty I like it. Maybe that's the caramel. Yeah yeah so I don't get

any citrus but I'm just you know I'm just average Joe. So yeah but I think

overall it's a good cup of coffee. How much did we pay for this? $5.99. Yeah

$5.99 it was on sale for $5.99 at

Winn-Dixie so for a twelve ounce bag. Yeah so overall I would buy this again

Yeah it's good. Yeah good. It's good drinkable coffee. It's nice that we both

agree on a coffee because sometimes there's a blend that I really like and

you're like. Yeah well that's it for this episode of Coffee Coffee Coffee. There

you go. Your average Joe coffee reviews. You

can find us on.. It's all you're gettin' for today.

...YouTube and also our website CoffeeCoffeeCoffeeShow.com and where else?

Instagram! Instagram. Follow us there. coffeecoffeecoffeeshow. Don't

just search coffeecoffeecoffee. Throw in that show on the end and we'll pop right up.

There ya go. Yeah okay have a good one. Cheers

For more infomation >> Gevalia House Blend Coffee Review - Duration: 3:45.

-------------------------------------------

How To Do Hot Stone Massage step by step - Duration: 5:08.

click on the subscribe button and press the bell icon or more updates

nation of warmed stones and massage techniques to relax tense muscles

relieve pain and stiffness and improve circulation the treatment can be used

for ailments like muscular aches arthritic conditions and autoimmune

disorders the heat of the stones penetrates the skin to promote better

blood flow release toxins and create a deeper muscle relaxation than in a

standard massage by placing the warmed stones over acupressure points you can

help release the flow of energy and promote the body's own healing process

those who do hot stone massage can also customize the treatment to a client's

specific needs and preferences

your materials

treatment are typically made of basalt due to their ability to retain heat the

stones should also be very smooth so they do not irritate the skin in any way

if you can't find basalt stones however smooth River rocks are fine you

can order a hot stone massage kit online from Amazon or Ebay however if you do

not want to buy your stones you can try going to a Roth quarry

you should have anywhere between twenty to thirty stones though some

professional massages may have upwards of 45 to 60 there should be at least two

large ovals around eight long to six wide seven stones you can fit in the

palm of your hand and eight small stones between the size of an egg or a quarter

to your area

if you do not have a table a bed where the floor will be fine

once you've chosen where the massage should take place you should lay out a

clean sheet or a thick towel for the person you are massaging to lay down on

this will not only help them be more comfortable but also help absorb any

excess oil from the massage to really create a relaxing environment try

lighting some Rimma therapy candles soothing scents like lavender lemongrass

eucalyptus and vanilla will help immerse your participant in the massage

experience you can also try playing some quiet classical music or rain sounds to

add to the mood

step three heat up to your stones

ideally you should prepare your stones 30 to 60 minutes before you begin your

massage the water should be around as the stones will likely cool down to 1

0-0 when taken out remember to wipe each stone down once you've removed it to

heat the stones use a crock-pot that can hold at least 6 quarts of water or a

large tabletop skillet that has sides close to three inches as you want the

stones to be around when you place them try cutting a candy thermometer in your

crock pot or skillet to monitor the temperature you should also keep the

crock pot setting to warm or low as you don't want the water to boil

you should also rub some massage oil on each stone before it's used

step four cover the participant if needed

should check with the participant to make sure they are comfortable with the

heat of the stones some people are going to react differently to different

temperatures and you don't want to burn them to prevent this it's best to place

a sheet or towel down and then put the stones on top of that keep in mind it

can take three to four minutes for the heat of the stones to penetrate to the

skin